Force and Motion

Newton's Laws of Motion

The study of Newton's laws of motion allows us to understand and accurately describe the motion of objects and the forces that act on those objects.

The basis for modern mechanics was developed in the seventeenth century by Sir Isaac Newton. From his studies of objects in motion, he formulated three fundamental laws.

Newton's first law of motion states "an object remains at rest (if originally at rest) or moves in a straight line with constant velocity if the net force on it is zero."

Newton's second law states "the acceleration of a body is proportional to the net (i.e., sum or resultant) force acting on it and in the direction of that net force." This law establishes the relationship between force, mass, and acceleration and can be written mathematically as shown in Equation 3-1.

where:

F	=	force (Newton = 1 kg-m/sec2, or lbf)
m	=	mass (kg or lbm)
a	=	acceleration (m/sec2 or ft/sec2)

This law is used to define force units and is one of the most important laws in physics. Also, Newton's first law is actually a consequence of this second law, since there is no acceleration when the force is zero, and the object is either at rest or moving with a constant velocity. Equation 3-1 can be used to calculate an objects weight at the surface of the earth. In this special case, F is the force, or weight, caused by the gravitational acceleration of the earth acting on the mass, m, of the object. When dealing with this type of problem, we designate the acceleration, g, which equals 9.8 m/sec² or 32.17 ft/sec² (g is called gravitational acceleration constant). Thus, equation 3-1 becomes F = mg for this case.

Newton's third law of motion states "if a body exerts a force on a second body, the second body exerts an equal and opposite force on the first." This law has also been stated as, "for every action there is an equal and opposite reaction."

The third law is basic to the understanding of force. It states that forces always occur in pairs of equal and opposite forces. Thus, the downward force exerted on a desk by a pencil is accompanied by an upward force of equal magnitude exerted on the pencil by the desk. This principle holds for all forces, variable or constant, regardless of their source.

One additional law attributed to Newton concerns mutual attractive forces between two bodies. It is known as the universal law of gravitation and is stated as follows.

"Each and every mass in the universe exerts a mutual, attractive gravitational force on every other mass in the universe. For any two masses, the force is directly proportional to the product of the two masses and is inversely proportional to the square of the distance between them."

Newton expressed the universal law of gravitation using Equation 3-2.

where:

F	=	force of attraction (Newton = 1 kg-m/sec2 or lbf)
G	=	universal constant of gravitation (6.673×10-11 m3/kg-sec2 or 3.44×10-8 lbm*ft2/slug2)
m1	=	mass of the first object (kg or lbm)
m2	=	mass of the second object (kg or lbm)
r	=	distance between the centers of the two objects (m or ft)

Using this universal law of gravitation, we can determine the value of g (gravitational acceleration constant), at the surface of the earth. We already know this value to be 9.8 m/sec² (or 32.17 ft/sec²), but it can be calculated using Equation 3-2.

Calculation:

First, we will assume that the earth is much larger than the object and that the object resides on the surface of the earth; therefore, the value of r will be equal to the radius of the earth. Second, we must understand that the force of attraction (F) in Equation 3-2 for the object is equal to the object's weight (F) as described in Equation 3.1. Setting these two equations equal to each other yields the following.

$$ F = G {M_e m_1 \over r^2} = m_1 a $$

where:

Me	=	mass of the earth (5.95×1024 kg)
m1	=	mass of the object
r	=	radius of the earth (6.367×106 m)

The mass (m₁) of the object cancels, and the value of (g) can be determined as follows since a = g by substituting (g) for (a) in the previous equation.

$$ g = G {M_e \over r^2} $$ $$ g = \left( 6.673 \times 10^{-11} { \text{m}^3 \over \text{kg}\cdot\text{sec}^2 } \right) \left({ 5.95 \times 10^{24} ~\text{kg} \over (6.367 \times 10^6 ~\text{m})^2 }\right) $$ $$ g = 9.8 {\text{m} \over \text{sec}^2} $$

If the object is a significant distance from the earth, we can demonstrate that (g) is not a constant value but varies with the distance (altitude) from the earth. If the object is at an altitude of 30 km (18.63 mi), then the value of (g) is as follows:

$$ g = \left( 6.673 \times 10^{-11} { \text{m}^3 \over \text{kg}\cdot\text{sec}^2 } \right) \left({ 5.95 \times 10^{24} ~\text{kg} \over (6.397 \times 10^6 ~\text{m})^2 }\right) $$ $$ g = 9.7 {\text{m} \over \text{sec}^2} $$

As you can see, a height of 30 km only changes (g) from 9.8 m/sec² to 9.7 m/sec². There will be an even smaller change for objects closer to the earth. Therefore, (g) is normally considered a constant value since most calculations involve objects close to the surface of the earth.

---

---

Momentum Principles

Momentum is a measure of the motion of a moving body. An understanding of momentum and the conservation of momentum provides essential tools in solving physics problems.

Momentum

Momentum is a basic and widely applicable concept of physics. It is, in a sense, the measure of the motion of a moving body. It is the result of the product of the body's mass and the velocity at which it is moving. Therefore, momentum can be defined using Equation 3-3.

where:

P	=	momentum of the object (kg-m/sec or ft-lbm/sec)
m	=	mass of the object (kg or lbm)
v	=	velocity of the object (m/sec or ft/sec)

Momentum is a vector quantity since it results from the velocity of the object. If different momentum quantities are to be added vectorially, the direction of each momentum must be taken into account. However, to simplify the understanding of momentum, only straight line motions will be considered.

Example:

Solution:

Force and Momentum

There is a direct relationship between force and momentum. The rate at which momentum changes with time is equal to the net force applied to an object. This relationship comes directly from Newton's second law of motion, F = ma. This is a special case of Newton's second law for a constant force which gives rise to a constant acceleration. The linking fact is that acceleration is the rate at which velocity changes with time. Therefore, we can determine the following:

We know that, F = ma

and since,

$$ a = {v - v_o \over t - t_o} $$

then,

which can also be written,

Substituting P for mv and P_o for mv_o,

$$ F = { P - P_o \over t - t_o } $$

or

From Equation 3-6, we can determine that force (F) is equal to the change in momentum per time.

Example:

Solution:

Conservation of Momentum

One of the most useful properties of momentum is that it is conserved. This means that if no net external force acts upon an object, the momentum of the object remains constant. Using Equation 3-6, we can see that if force (F) is equal to zero, then ΔP = 0. It is most important for collisions, explosions, etc., where the external force is negligible, and states that the momentum before the event (collision, explosion) equals the momentum following the event.

The conservation of momentum applies when a bullet is fired from a gun. Prior to firing the gun, both the gun and the bullet are at rest (i.e., V_G and V_B are zero), and therefore the total momentum is zero. This can be written as follows:

or

When the gun is fired, the momentum of the recoiling gun is equal and opposite to the momentum of the bullet. That is, the momentum of the bullet (m_Bv_B) is equal to the momentum of the gun (m_Gv_G), but of opposite direction.

The development of the law of conservation of momentum does not consider whether the collision is elastic or inelastic. In an elastic collision, both momentum and kinetic energy (i.e., energy due to an objects velocity) are conserved. A common example of an elastic collision is the head-on collision of two billiard balls of equal mass. In an inelastic collision, momentum is conserved, but system kinetic energy is not conserved. An example of an inelastic collision is the head-on collision of two automobiles where part of the initial kinetic energy is lost as the metal crumples during the impact. The concept of kinetic energy will be discussed further in Module 5 of this course.

The law of conservation of momentum can be mathematically expressed in several different ways. In general, it can be stated that the sum of a system's initial momentum is equal to the sum of a system's final momentum.

In the case where a collision of two objects occurs, the conservation of momentum can be stated as follows.

or

In the case where two bodies collide and have identical final velocities, equation 3-10 applies.

For example, consider two railroad cars rolling on a level, frictionless track (see Figure 1). The cars collide, become coupled, and roll together at a final velocity (v_f). The momentum before and after the collision is expressed with Equation 3-10.

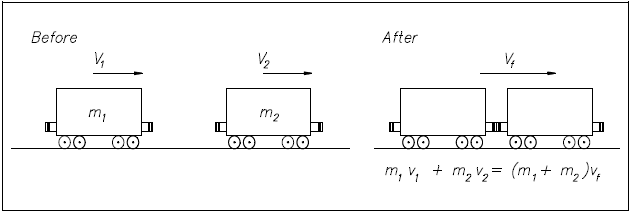
If the initial velocities of the two objects (v₁ and v₂) are known, then the final velocity (v_f) can be calculated by rearranging Equation 3-10 into Equation 3-11.

Example:

Solution:

---

---

---