Properties of Metals
This page provides the chapters on the properties of metals from the "DOE Fundamentals Handbook: Material Science," DOE-HDBK-1017/1-93, U.S. Department of Energy, Jan 1993.
Other related chapters from the "DOE Fundamentals Handbook: Material Science" can be seen to the right.
- Structure of Metals
- Properties of Metals
- Material Selection
- Thermal Stress
- Brittle Fracture
Stress
Any component, no matter how simple or complex, has to transmit or sustain a mechanical load of some sort. The load may be one of the following types: a load that is applied steadily ("dead" load); a load that fluctuates, with slow or fast changes in magnitude ("live" load); a load that is applied suddenly (shock load); or a load due to impact in some form. Stress is a form of load that may be applied to a component. Personnel need to be aware how stress may be applied and how it effects the component.
Definition of Stress
When a metal is subjected to a load (force), it is distorted or deformed, no matter how strong the metal or light the load. If the load is small, the distortion will probably disappear when the load is removed. The intensity, or degree, of distortion is known as strain. If the distortion disappears and the metal returns to its original dimensions upon removal of the load, the strain is called elastic strain. If the distortion disappears and the metal remains distorted, the strain type is called plastic strain. Strain will be discussed in more detail in the next chapter.
When a load is applied to metal, the atomic structure itself is strained, being compressed, warped or extended in the process. The atoms comprising a metal are arranged in a certain geometric pattern, specific for that particular metal or alloy, and are maintained in that pattern by interatomic forces. When so arranged, the atoms are in their state of minimum energy and tend to remain in that arrangement. Work must be done on the metal (that is, energy must be added) to distort the atomic pattern. (Work is equal to force times the distance the force moves.)
Stress is the internal resistance, or counterforce, of a material to the distorting effects of an external force or load. These counterforces tend to return the atoms to their normal positions. The total resistance developed is equal to the external load. This resistance is known as stress.
Although it is impossible to measure the intensity of this stress, the external load and the area to which it is applied can be measured. Stress (σ) can be equated to the load per unit area or the force (F) applied per cross-sectional area (A) perpendicular to the force as shown in Equation (2-1).
where:
| σ = stress (psi or lbs of force per in.2) |
| F = applied force (lbs of force per in.2) |
| A = cross-sectional area (in.2) |
Types of Stress
Stresses occur in any material that is subject to a load or any applied force. There are many types of stresses, but they can all be generally classified in one of six categories: residual stresses, structural stresses, pressure stresses, flow stresses, thermal stresses, and fatigue stresses.
Residual Stresses
Residual stresses are due to the manufacturing processes that leave stresses in a material. Welding leaves residual stresses in the metals welded. Stresses associated with welding are further discussed later in this module.
Structural Stresses
Structural stresses are stresses produced in structural members because of the weights they support. The weights provide the loadings. These stresses are found in building foundations and frameworks, as well as in machinery parts.
Pressure Stresses
Pressure stresses are stresses induced in vessels containing pressurized materials. The loading is provided by the same force producing the pressure. In a reactor facility, the reactor vessel is a prime example of a pressure vessel.
Flow Stresses
Flow stresses occur when a mass of flowing fluid induces a dynamic pressure on a conduit wall. The force of the fluid striking the wall acts as the load. This type of stress may be applied in an unsteady fashion when flow rates fluctuate. Water hammer is an example of a transient flow stress.
Thermal Stresses
Thermal stresses exist whenever temperature gradients are present in a material. Different temperatures produce different expansions and subject materials to internal stress. This type of stress is particularly noticeable in mechanisms operating at high temperatures that are cooled by a cold fluid. Thermal stress is further discussed in Module 3.
Fatigue Stresses
Fatigue stresses are due to cyclic application of a stress. The stresses could be due to vibration or thermal cycling. Fatigue stresses are further discussed in Module 4.
The importance of all stresses is increased when the materials supporting them are flawed. Flaws tend to add additional stress to a material. Also, when loadings are cyclic or unsteady, stresses can affect a material more severely. The additional stresses associated with flaws and cyclic loading may exceed the stress necessary for a material to fail.
Types of Applied Stress
Stress intensity within the body of a component is expressed as one of three basic types of internal load. They are known as tensile, compressive, and shear. Figure 1 illustrates the different types of stress. Mathematically, there are only two types of internal load because tensile and compressive stress may be regarded as the positive and negative versions of the same type of normal loading.
However, in mechanical design, the response of components to the two conditions can be so different that it is better, and safer, to regard them as separate types.
As illustrated in Figure 1, the plane of a tensile or compressive stress lies perpendicular to the axis of operation of the force from which it originates. The plane of a shear stress lies in the plane of the force system from which it originates. It is essential to keep these differences quite clear both in mind and mode of expression.
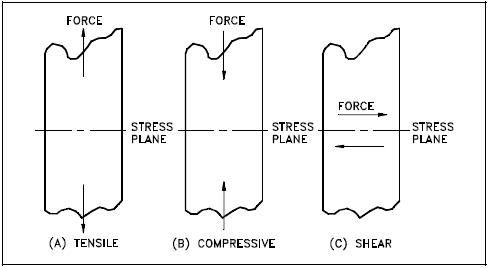
Tensile Stress
Tensile stress is that type of stress in which the two sections of material on either side of a stress plane tend to pull apart or elongate as illustrated in Figure 1(a).
Compressive Stress
Compressive stress is the reverse of tensile stress. Adjacent parts of the material tend to press against each other through a typical stress plane as illustrated in Figure 1(b).
Shear Stress
Shear stress exists when two parts of a material tend to slide across each other in any typical plane of shear upon application of force parallel to that plane as illustrated in Figure 1(c).
Assessment of mechanical properties is made by addressing the three basic stress types. Because tensile and compressive loads produce stresses that act across a plane, in a direction perpendicular (normal) to the plane, tensile and compressive stresses are called normal stresses. The shorthand designations are as follows.
- For tensile stresses: "+SN" (or "SN") or "σ" (sigma)
- For compressive stresses: "−SN" or "−σ" (minus sigma)
The ability of a material to react to compressive stress or pressure is called compressibility. For example, metals and liquids are incompressible, but gases and vapors are compressible. The shear stress is equal to the force divided by the area of the face parallel to the direction in which the force acts, as shown in Figure 1(c).
Two types of stress can be present simultaneously in one plane, provided that one of the stresses is shear stress. Under certain conditions, different basic stress type combinations may be simultaneously present in the material. An example would be a reactor vessel during operation. The wall has tensile stress at various locations due to the temperature and pressure of the fluid acting on the wall. Compressive stress is applied from the outside at other locations on the wall due to outside pressure, temperature, and constriction of the supports associated with the vessel. In this situation, the tensile and compressive stresses are considered principal stresses. If present, shear stress will act at a 90° angle to the principal stress.
We have a number of structural calculators to choose from. Here are just a few:
Strain
When stress is present strain will be involved also. The two types of strain will be discussed in this chapter. Personnel need to be aware how strain may be applied and how it affects the component.
Definition of Strain
In the use of metal for mechanical engineering purposes, a given state of stress usually exists in a considerable volume of the material. Reaction of the atomic structure will manifest itself on a macroscopic scale. Therefore, whenever a stress (no matter how small) is applied to a metal, a proportional dimensional change or distortion must take place.
Such a proportional dimensional change (intensity or degree of the distortion) is called strain and is measured as the total elongation per unit length of material due to some applied stress. Equation 2-2 illustrates this proportion or distortion.
where:
| ε = strain (in./in.) |
| δ = total elongation (in.) |
| L = original length (in.) |
Types of Strain
Strain may take two forms; elastic strain and plastic deformation.
Elastic Strain
Elastic strain is a transitory dimensional change that exists only while the initiating stress is applied and disappears immediately upon removal of the stress. Elastic strain is also called elastic deformation. The applied stresses cause the atoms in a crystal to move from their equilibrium position. All the atoms are displaced the same amount and still maintain their relative geometry. When the stresses are removed, all the atoms return to their original positions and no permanent deformation occurs.
Plastic Deformation
Plastic deformation (or plastic strain) is a dimensional change that does not disappear when the initiating stress is removed. It is usually accompanied by some elastic strain.
The phenomenon of elastic strain and plastic deformation in a material are called elasticity and plasticity, respectively.
At room temperature, most metals have some elasticity, which manifests itself as soon as the slightest stress is applied. Usually, they also possess some plasticity, but this may not become apparent until the stress has been raised appreciably. The magnitude of plastic strain, when it does appear, is likely to be much greater than that of the elastic strain for a given stress increment. Metals are likely to exhibit less elasticity and more plasticity at elevated temperatures. A few pure unalloyed metals (notably aluminum, copper and gold) show little, if any, elasticity when stressed in the annealed (heated and then cooled slowly to prevent brittleness) condition at room temperature, but do exhibit marked plasticity. Some unalloyed metals and many alloys have marked elasticity at room temperature, but no plasticity.
The state of stress just before plastic strain begins to appear is known as the proportional limit, or elastic limit, and is defined by the stress level and the corresponding value of elastic strain. The proportional limit is expressed in pounds per square inch. For load intensities beyond the proportional limit, the deformation consists of both elastic and plastic strains.
As mentioned previously in this chapter, strain measures the proportional dimensional change with no load applied. Such values of strain are easily determined and only cease to be sufficiently accurate when plastic strain becomes dominant.
When metal experiences strain, its volume remains constant. Therefore, if volume remains constant as the dimension changes on one axis, then the dimensions of at least one other axis must change also. If one dimension increases, another must decrease. There are a few exceptions. For example, strain hardening involves the absorption of strain energy in the material structure, which results in an increase in one dimension without an offsetting decrease in other dimensions. This causes the density of the material to decrease and the volume to increase.
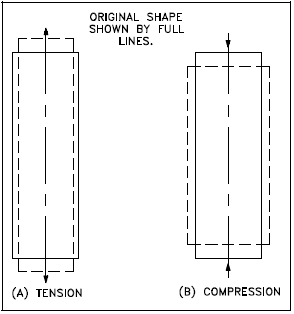
If a tensile load is applied to a material, the material will elongate on the axis of the load (perpendicular to the tensile stress plane), as illustrated in Figure 2(a). Conversely, if the load is compressive, the axial dimension will decrease, as illustrated in Figure 2(b). If volume is constant, a corresponding lateral contraction or expansion must occur. This lateral change will bear a fixed relationship to the axial strain. The relationship, or ratio, of lateral to axial strain is called Poisson's ratio after the name of its discoverer. It is usually symbolized by ν.
Deformation of Cubic Structures
Whether or not a material can deform plastically at low applied stresses depends on its lattice structure. It is easier for planes of atoms to slide by each other if those planes are closely packed. Therefore lattice structures with closely packed planes allow more plastic deformation than those that are not closely packed. Also, cubic lattice structures allow slippage to occur more easily than non-cubic lattices. This is because of their symmetry which provides closely packed planes in several directions. Most metals are made of the body-centered cubic (BCC), face-centered cubic (FCC), or hexagonal close-packed (HCP) crystals, discussed in more detail in the Module 1, Structure of Metals. A face-centered cubic crystal structure will deform more readily under load before breaking than a body-centered cubic structure.
The BCC lattice, although cubic, is not closely packed and forms strong metals. α-iron and tungsten have the BCC form. The FCC lattice is both cubic and closely packed and forms more ductile materials. γ-iron, silver, gold, and lead are FCC structured. Finally, HCP lattices are closely packed, but not cubic. HCP metals like cobalt and zinc are not as ductile as the FCC metals.
Young's Modulus
This chapter discusses the mathematical method used to calculate the elongation of a material under tensile force and elasticity of a material.
Hooke's Law
If a metal is lightly stressed, a temporary deformation, presumably permitted by an elastic displacement of the atoms in the space lattice, takes place. Removal of the stress results in a gradual return of the metal to its original shape and dimensions. In 1678 an English scientist named Robert Hooke ran experiments that provided data that showed that in the elastic range of a material, strain is proportional to stress. The elongation of the bar is directly proportional to the tensile force and the length of the bar and inversely proportional to the cross-sectional area and the modulus of elasticity.
Hooke's experimental law may be given by Equation (2-3).
This simple linear relationship between the force (stress) and the elongation (strain) was formulated using the following notation.
| P = | force producing extension of bar (lbf) |
| L = | length of bar (in.) |
| A = | cross-sectional area of bar (in.2) |
| δ = | total elongation of bar (in.) |
| E = | elastic constant of the material, called the Modulus of Elasticity, or Young's Modulus (lbf/in.2) |
The quantity E, the ratio of the unit stress to the unit strain, is the modulus of elasticity of the material in tension or compression and is often called Young's Modulus.
Previously, we learned that tensile stress, or simply stress, was equated to the load per unit area or force applied per cross-sectional area perpendicular to the force measured in pounds force per square inch.
We also learned that tensile strain, or the elongation of a bar per unit length, is determined by:
Thus, the conditions of the experiment described above are adequately expressed by Hooke's Law for elastic materials. For materials under tension, strain (ε) is proportional to applied stress σ.
where
| E = Young's Modulus (lbf/in.2) |
| σ = stress (psi) |
| ε = strain (in./in.) |
Young's Modulus (Elastic Modulus)
Young's Modulus (sometimes referred to as Modulus of Elasticity, meaning "measure" of elasticity) is an extremely important characteristic of a material. It is the numerical evaluation of Hooke's Law, namely the ratio of stress to strain (the measure of resistance to elastic deformation). To calculate Young's Modulus, stress (at any point) below the proportional limit is divided by corresponding strain. It can also be calculated as the slope of the straight-line portion of the stress-strain curve. (The positioning on a stress-strain curve will be discussed later.)
$$ E = \text{Elastic Modulus} = { \text{stress} \over \text{strain} } = { \text{psi} \over \text{in./in.} } = \text{psi} $$or
We can now see that Young's Modulus may be easily calculated, provided that the stress and corresponding unit elongation or strain have been determined by a tensile test as described previously. Strain (ε) is a number representing a ratio of two lengths; therefore, we can conclude that the Young's Modulus is measured in the same units as stress (σ), that is, in pounds per square inch. Table 1 gives average values of the Modulus E for several metals used in DOE facilities construction. Yield strength and ultimate strength will be discussed in more detail in the next chapter.
| E (psi) | Yield Strength (psi) | Ultimate Strength (psi) | |
|---|---|---|---|
| Aluminum | 1.0×107 | 3.5×104 to 4.5×104 | 5.4×104 to 6.5×104 |
| Stainless Steel | 2.9×107 | 4.0×104 to 5.0×104 | 7.8×104 to 10×104 |
| Carbon Steel | 3.0×107 | 3.0×104 to 4.0×104 | 5.5×104 to 6.5×104 |
Example:
What is the elongation of 200 in. of aluminum wire with a 0.01 square in. area if it supports a weight of 100 lb?
Solution:
We have a number of structural calculators to choose from. Here are just a few:
Stress-Strain Relationship
Most polycrystalline materials have within their elastic range an almost constant relationship between stress and strain. Experiments by an English scientist named Robert Hooke led to the formation of Hooke's Law, which states that in the elastic range of a material strain is proportional to stress. The ratio of stress to strain, or the gradient of the stress-strain graph, is called the Young's Modulus.
Elastic Moduli
The elastic moduli relevant to polycrystalline material are Young's Modulus of Elasticity, the Shear Modulus of Elasticity, and the Bulk Modulus of Elasticity.
Young's Modulus
Young's Modulus of Elasticity is the elastic modulus for tensile and compressive stress and is usually assessed by tensile tests. Young's Modulus of Elasticity is discussed in detail in the preceding chapter.
Shear Modulus
The Shear Modulus of Elasticity is derived from the torsion of a cylindrical test piece. Its symbol is G.
Bulk Modulus
The Bulk Modulus of Elasticity is the elastic response to hydrostatic pressure and equilateral tension or the volumetric response to hydrostatic pressure and equilateral tension. It is also the property of a material that determines the elastic response to the application of stress.
Tensile (Load) Tests and Stress-Strain Curves
To determine the load-carrying ability and the amount of deformation before fracture, a sample of material is commonly tested by a Tensile Test. This test consists of applying a gradually increasing force of tension at one end of a sample length of the material. The other end is anchored in a rigid support so that the sample is slowly pulled apart. The testing machine is equipped with a device to indicate, and possibly record, the magnitude of the force throughout the test. Simultaneous measurements are made of the increasing length of a selected portion at the middle of the specimen, called the gage length. The measurements of both load and elongation are ordinarily discontinued shortly after plastic deformation begins; however, the maximum load reached is always recorded. Fracture point is the point where the material fractures due to plastic deformation. After the specimen has been pulled apart and removed from the machine, the fractured ends are fitted together and measurements are made of the now-extended gage length and of the average diameter of the minimum cross section. The average diameter of the minimum cross section is measured only if the specimen used is cylindrical.
The tabulated results at the end of the test consist of the following.
- designation of the material under test
- original cross section dimensions of the specimen within the gage length
- original gage length
- a series of frequent readings identifying the load and the corresponding gage length dimension
- final average diameter of the minimum cross section
- final gage length
- description of the appearance of the fracture surfaces (for example, cup-cone, wolf's ear, diagonal, start)
A graph of the results is made from the tabulated data. Some testing machines are equipped with an autographic attachment that draws the graph during the test. (The operator need not record any load or elongation readings except the maximum for each.) The coordinate axes of the graph are strain for the x-axis or scale of abscissae, and stress for the y-axis or scale of ordinates. The ordinate for each point plotted on the graph is found by dividing each of the tabulated loads by the original cross-sectional area of the sample; the corresponding abscissa of each point is found by dividing the increase in gage length by the original gage length. These two calculations are made as follows.
Stress and strain, as computed here, are sometimes called "engineering stress and strain." They are not true stress and strain, which can be computed on the basis of the area and the gage length that exist for each increment of load and deformation. For example, true strain is the natural log of the elongation ( ln(L/Lo) ), and true stress is P/A, where A is area. The latter values are usually used for scientific investigations, but the engineering values are useful for determining the load-carrying values of a material. Below the elastic limit, engineering stress and true stress are almost identical.
The graphic results, or stress-strain diagram, of a typical tension test for structural steel is shown in Figure 3. The ratio of stress to strain, or the gradient of the stress-strain graph, is called the Modulus of Elasticity or Elastic Modulus. The slope of the portion of the curve where stress is proportional to strain (between Points 1 and 2) is referred to as Young's Modulus and Hooke's Law applies.
The following observations are illustrated in Figure 3:
- Hooke's Law applies between Points 1 and 2.
- Hooke's Law becomes questionable between Points 2 and 3 and strain increases more rapidly.
- The area between Points 1 and 2 is called the elastic region. If stress is removed, the material will return to its original length.
- Point 2 is the proportional limit (PL) or elastic limit, and Point 3 is the yield strength (YS) or yield point.
- The area between Points 2 and 5 is known as the plastic region because the material will not return to its original length.
- Point 4 is the point of ultimate strength and Point 5 is the fracture point at which failure of the material occurs.
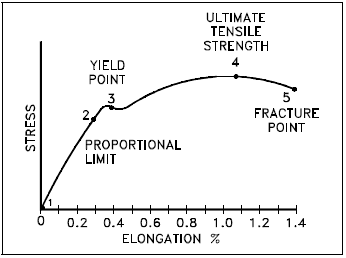
Figure 3 is a stress-strain curve typical of a ductile material where the strength is small, and the plastic region is great. The material will bear more strain (deformation) before fracture.
Figure 4 is a stress-strain curve typical of a brittle material where the plastic region is small and the strength of the material is high.
The tensile test supplies three descriptive facts about a material. These are the stress at which observable plastic deformation or "yielding" begins; the ultimate tensile strength or maximum intensity of load that can be carried in tension; and the percent elongation or strain (the amount the material will stretch) and the accompanying percent reduction of the cross-sectional area caused by stretching. The rupture or fracture point can also be determined.
We have a number of structural calculators to choose from. Here are just a few:
Physical Properties
Material is selected for various applications in a reactor facility based on its physical and chemical properties. This chapter discusses the physical properties of material. Appendix A contains a discussion on the compatibility of tritium with various materials.
Strength
Strength is the ability of a material to resist deformation. The strength of a component is usually considered based on the maximum load that can be borne before failure is apparent. If under simple tension the permanent deformation (plastic strain) that takes place in a component before failure, the load-carrying capacity, at the instant of final rupture, will probably be less than the maximum load supported at a lower strain because the load is being applied over a significantly smaller cross-sectional area. Under simple compression, the load at fracture will be the maximum applicable over a significantly enlarged area compared with the cross-sectional area under no load.
This obscurity can be overcome by utilizing a nominal stress figure for tension and shear. This is found by dividing the relevant maximum load by the original area of cross section of the component. Thus, the strength of a material is the maximum nominal stress it can sustain. The nominal stress is referred to in quoting the "strength" of a material and is always qualified by the type of stress, such as tensile strength, compressive strength, or shear strength.
For most structural materials, the difficulty in finding compressive strength can be overcome by substituting the tensile strength value for compressive strength. This substitution is a safe assumption since the nominal compression strength is always greater than the nominal tensile strength because the effective cross section increases in compression and decreases in tension.
When a force is applied to a metal, layers of atoms within the crystal structure move in relation to adjacent layers of atoms. This process is referred to as slip. Grain boundaries tend to prevent slip. The smaller the grain size, the larger the grain boundary area. Decreasing the grain size through cold or hot working of the metal tends to retard slip and thus increases the strength of the metal. Cold and hot working are discussed in the next chapter.
Ultimate Tensile Strength
The ultimate tensile strength (UTS) is the maximum resistance to fracture. It is equivalent to the maximum load that can be carried by one square inch of cross-sectional area when the load is applied as simple tension. It is expressed in pounds per square inch.
If the complete engineering stress-strain curve is available, as shown in Figure 3, the ultimate tensile strength appears as the stress coordinate value of the highest point on the curve. Materials that elongate greatly before breaking undergo such a large reduction of cross-sectional area that the material will carry less load in the final stages of the test (this was noted in Figure 3 and Figure 4 by the decrease in stress just prior to rupture). A marked decrease in cross-section is called "necking." Ultimate tensile strength is often shortened to "tensile strength" or even to "the ultimate." "Ultimate strength" is sometimes used but can be misleading and, therefore, is not used in some disciplines.
Yield Strength
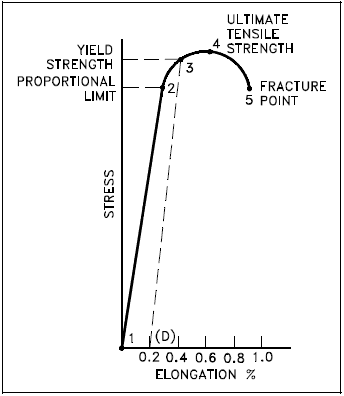
A number of terms have been defined for the purpose of identifying the stress at which plastic deformation begins. The value most commonly used for this purpose is the yield strength. The yield strength is defined as the stress at which a predetermined amount of permanent deformation occurs. The graphical portion of the early stages of a tension test is used to evaluate yield strength. To find yield strength, the predetermined amount of permanent strain is set along the strain axis of the graph, to the right of the origin (zero). It is indicated in Figure 5 as Point (D).
A straight line is drawn through Point (D) at the same slope as the initial portion of the stress-strain curve. The point of intersection of the new line and the stress-strain curve is projected to the stress axis. The stress value, in pounds per square inch, is the yield strength. It is indicated in Figure 5 as Point 3. This method of plotting is done for the purpose of subtracting the elastic strain from the total strain, leaving the predetermined "permanent offset" as a remainder. When yield strength is reported, the amount of offset used in the determination should be stated. For example, "Yield Strength (at 0.2% offset) = 51,200 psi."

Examples of Yield Strength
Some examples of yield strength for metals are as follows.
| Aluminum | 3.5×104 to 4.5×104 psi |
| Stainless steel | 4.0×104 to 5.0×104 psi |
| Carbon steel | 3.0×104 to 4.0×104 psi |
Alternate Values
Alternate values are sometimes used instead of yield strength. Several of these are briefly described below.
- The yield point, determined by the divider method, involves an observer with a pair of dividers watching for visible elongation between two gage marks on the specimen. When visible stretch occurs, the load at that instant is recorded, and the stress corresponding to that load is calculated.
- Soft steel, when tested in tension, frequently displays a peculiar characteristic, known as a yield point. If the stress-strain curve is plotted, a drop in the load (or sometimes a constant load) is observed although the strain continues to increase. Eventually, the metal is strengthened by the deformation, and the load increases with further straining. The high point on the S-shaped portion of the curve, where yielding began, is known as the upper yield point, and the minimum point is the lower yield point. This phenomenon is very troublesome in certain deep drawing operations of sheet steel. The steel continues to elongate and to become thinner at local areas where the plastic strain initiates, leaving unsightly depressions called stretcher strains or "worms."
- The proportional limit is defined as the stress at which the stress-strain curve first deviates from a straight line. Below this limiting value of stress, the ratio of stress to strain is constant, and the material is said to obey Hooke's Law (stress is proportional to strain). The proportional limit usually is not used in specifications because the deviation begins so gradually that controversies are sure to arise as to the exact stress at which the line begins to curve.
- The elastic limit has previously been defined as the stress at which plastic deformation begins. This limit cannot be determined from the stress-strain curve. The method of determining the limit would have to include a succession of slightly increasing loads with intervening complete unloading for the detection of the first plastic deformation or "permanent set." Like the proportional limit, its determination would result in controversy. Elastic limit is used, however, as a descriptive, qualitative term.
In many situations, the yield strength is used to identify the allowable stress to which a material can be subjected. For components that have to withstand high pressures, such as those used in pressurized water reactors (PWRs), this criterion is not adequate. To cover these situations, the maximum shear stress theory of failure has been incorporated into the ASME (The American Society of Mechanical Engineers) Boiler and Pressure Vessel Code, Section III, Rules for Construction of Nuclear Pressure Vessels. The maximum shear stress theory of failure was originally proposed for use in the U.S. Naval Reactor Program for PWRs. It will not be discussed in this text.
Ductility
The percent elongation reported in a tensile test is defined as the maximum elongation of the gage length divided by the original gage length. The measurement is determined as shown in Figure 6.
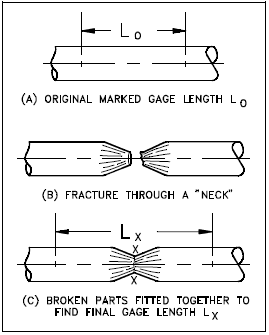
Reduction of area is the proportional reduction of the cross-sectional area of a tensile test piece at the plane of fracture measured after fracture.
The reduction of area is reported as additional information (to the percent elongation) on the deformational characteristics of the material. The two are used as indicators of ductility, the ability of a material to be elongated in tension. Because the elongation is not uniform over the entire gage length and is greatest at the center of the neck, the percent elongation is not an absolute measure of ductility. (Because of this, the gage length must always be stated when the percent elongation is reported.) The reduction of area, being measured at the minimum diameter of the neck, is a better indicator of ductility.
Ductility is more commonly defined as the ability of a material to deform easily upon the application of a tensile force, or as the ability of a material to withstand plastic deformation without rupture. Ductility may also be thought of in terms of bendability and crushability. Ductile materials show large deformation before fracture. The lack of ductility is often termed brittleness. Usually, if two materials have the same strength and hardness, the one that has the higher ductility is more desirable. The ductility of many metals can change if conditions are altered. An increase in temperature will increase ductility. A decrease in temperature will cause a decrease in ductility and a change from ductile to brittle behavior. Irradiation will also decrease ductility, as discussed in Module 5.
Cold-working also tends to make metals less ductile. Cold-working is performed in a temperature region and over a time interval to obtain plastic deformation, but not relieving the strain hardening. Minor additions of impurities to metals, either deliberate or unintentional, can have a marked effect on the change from ductile to brittle behavior. The heating of a cold-worked metal to or above the temperature at which metal atoms return to their equilibrium positions will increase the ductility of that metal. This process is called annealing.
Ductility is desirable in the high temperature and high pressure applications in reactor plants because of the added stresses on the metals. High ductility in these applications helps prevent brittle fracture, which is discussed in Module 4.
Malleability
Where ductility is the ability of a material to deform easily upon the application of a tensile force, malleability is the ability of a metal to exhibit large deformation or plastic response when being subjected to compressive force. Uniform compressive force causes deformation in the manner shown in Figure 7. The material contracts axially with the force and expands laterally. Restraint due to friction at the contact faces induces axial tension on the outside. Tensile forces operate around the circumference with the lateral expansion or increasing girth. Plastic flow at the center of the material also induces tension.
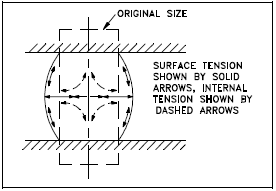
Therefore, the criterion of fracture (that is, the limit of plastic deformation) for a plastic material is likely to depend on tensile rather than compressive stress. Temperature change may modify both the plastic flow mode and the fracture mode.
Toughness
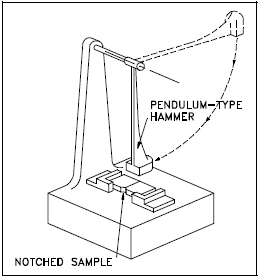
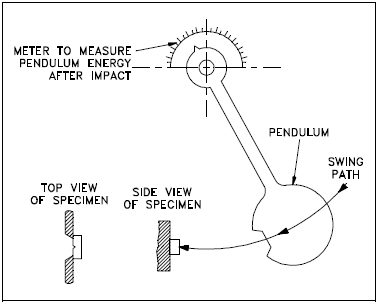
The quality known as toughness describes the way a material reacts under sudden impacts. It is defined as the work required to deform one cubic inch of metal until it fractures. Toughness is measured by the Charpy test or the Izod test. Both of these tests use a notched sample. The location and shape of the notch are standard. The points of support of the sample, as well as the impact of the hammer, must bear a constant relationship to the location of the notch. The tests are conducted by mounting the samples as shown in Figure 8 and allowing a pendulum of a known weight to fall from a set height. The maximum energy developed by the hammer is 120 ft-lb in the Izod test and 240 ft-lb in the Charpy test. By properly calibrating the machine, the energy absorbed by the specimen may be measured from the upward swing of the pendulum after it has fractured the material specimen as shown in Figure 9. The greater the amount of energy absorbed by the specimen, the smaller the upward swing of the pendulum will be and the tougher the material is.
Indication of toughness is relative and applicable only to cases involving exactly this type of sample and method of loading. A sample of a different shape will yield an entirely different result. Notches confine the deformation to a small volume of metal that reduces toughness. In effect, it is the shape of the metal in addition to the material composition that determines the toughness of the material.
Hardness
Hardness is the property of a material that enables it to resist plastic deformation, penetration, indentation, and scratching. Therefore, hardness is important from an engineering standpoint because resistance to wear by either friction or erosion by steam, oil, and water generally increases with hardness.
Hardness tests serve an important need in industry even though they do not measure a unique quality that can be termed hardness. The tests are empirical, based on experiments and observation, rather than fundamental theory. Its chief value is as an inspection device, able to detect certain differences in material when they arise even though these differences may be undefinable. For example, two lots of material that have the same hardness may or may not be alike, but if their hardness is different, the materials certainly are not alike.
Several methods have been developed for hardness testing. Those most often used are Brinell, Rockwell, Vickers, Tukon, Sclerscope, and the files test. The first four are based on indentation tests and the fifth on the rebound height of a diamond-tipped metallic hammer. The file test establishes the characteristics of how well a file takes a bite on the material.
As a result of many tests, comparisons have been prepared using formulas, tables, and graphs that show the relationships between the results of various hardness tests of specific alloys. There is, however, no exact mathematical relation between any two of the methods. For this reason, the result of one type of hardness test converted to readings of another type should carry the notation "_____ converted from _____" (for example "352 Brinell converted from Rockwell C-38").
Another convenient conversion is that of Brinell hardness to ultimate tensile strength. For quenched and tempered steel, the tensile strength (psi) is about 500 times the Brinell hardness number (provided the strength is not over 200,000 psi).
How Alloys Affect Physical Properties
Nickel is an important alloying element. In concentrations of less than 5%, nickel will raise the toughness and ductility of steel without raising the hardness. It will not raise the hardness when added in these small quantities because it does not form carbides, solid compounds with carbon.
Chromium in steel forms a carbide that hardens the metal. The chromium atoms may also occupy locations in the crystal lattice, which will have the effect of increasing hardness without affecting ductility. The addition of nickel intensifies the effects of chromium, producing a steel with increased hardness and ductility.
Copper is quite similar to nickel in its effects on steel. Copper does not form a carbide, but increases hardness by retarding dislocation movement.
Molybdenum forms a complex carbide when added to steel. Because of the structure of the carbide, it hardens steel substantially, but also minimizes grain enlargement. Molybdenum tends to augment the desirable properties of both nickel and chromium.
Stainless steels are alloy steels containing at least 12% chromium. An important characteristic of these steels is their resistance to many corrosive conditions.
We have a number of structural calculators to choose from. Here are just a few:
Working of Metals
Heat treatment and working of the metal are discussed as metallurgical processes used to change the properties of metals. Personnel need to understand the effects on metals to select the proper material for a reactor facility.
Heat Treatment
Heat treatment of large carbon steel components is done to take advantage of crystalline defects and their effects and thus obtain certain desirable properties or conditions.
During manufacture, by varying the rate of cooling (quenching) of the metal, grain size and grain patterns are controlled. Grain characteristics are controlled to produce different levels of hardness and tensile strength. Generally, the faster a metal is cooled, the smaller the grain sizes will be. This will make the metal harder. As hardness and tensile strength increase in heat-treated steel, toughness and ductility decrease.
The cooling rate used in quenching depends on the method of cooling and the size of the metal. Uniform cooling is important to prevent distortion. Typically, steel components are quenched in oil or water.
Because of the crystal pattern of type 304 stainless steel in the reactor tank (tritium production facility), heat treatment is unsuitable for increasing the hardness and strength.
Welding can induce internal stresses that will remain in the material after the welding is completed. In stainless steels, such as type 304, the crystal lattice is face-centered cubic (austenite). During high temperature welding, some surrounding metal may be elevated to between 500°F and 1000°F. In this temperature region, the austenite is transformed into a body-centered cubic lattice structure (bainite). When the metal has cooled, regions surrounding the weld contain some original austenite and some newly formed bainite. A problem arises because the "packing factor" (PF = volume of atoms/volume of unit cell) is not the same for FCC crystals as for BCC crystals.
The bainite that has been formed occupies more space than the original austenite lattice. This elongation of the material causes residual compressive and tensile stresses in the material. Welding stresses can be minimized by using heat sink welding, which results in lower metal temperatures, and by annealing.
Annealing is another common heat treating process for carbon steel components. During annealing, the component is heated slowly to an elevated temperature and held there for a long period of time, then cooled. The annealing process is done to obtain the following effects.
- to soften the steel and improve ductility
- to relieve internal stresses caused by previous processes such as heat treatment, welding, or machining
- to refine the grain structure
Cold and Hot Working
Plastic deformation which is carried out in a temperature region and over a time interval such that the strain hardening is not relieved is called cold work. Considerable knowledge on the structure of the cold-worked state has been obtained. In the early stages of plastic deformation, slip is essentially on primary glide planes and the dislocations form coplanar arrays. As deformation proceeds, cross slip takes place. The cold-worked structure forms high dislocation density regions that soon develop into networks. The grain size decreases with strain at low deformation but soon reaches a fixed size. Cold working will decrease ductility.
Hot working refers to the process where metals are deformed above their recrystallization temperature and strain hardening does not occur. Hot working is usually performed at elevated temperatures. Lead, however, is hot-worked at room temperature because of its low melting temperature. At the other extreme, molybdenum is cold-worked when deformed even at red heat because of its high recrystallization temperature.
The resistance of metals to plastic deformation generally falls with temperature. For this reason, larger massive sections are always worked hot by forging, rolling, or extrusion. Metals display distinctly viscous characteristics at sufficiently high temperatures, and their resistance to flow increases at high forming rates. This occurs not only because it is a characteristic of viscous substances, but because the rate of recrystallization may not be fast enough.
Corrosion
Corrosion is a major factor in the selection of material for a reactor plant. The material selected must resist the various types of corrosion discussed in the Chemistry Fundamentals Handbook.
Corrosion
Corrosion is the deterioration of a material due to interaction with its environment. It is the process in which metallic atoms leave the metal or form compounds in the presence of water and gases. Metal atoms are removed from a structural element until it fails, or oxides build up inside a pipe until it is plugged. All metals and alloys are subject to corrosion. Even the noble metals, such as gold, are subject to corrosive attack in some environments.
The corrosion of metals is a natural process. Most metals are not thermodynamically stable in the metallic form; they want to corrode and revert to the more stable forms that are normally found in ores, such as oxides. Corrosion is of primary concern in nuclear reactor plants. Corrosion occurs continuously throughout the reactor plant, and every metal is subject to it. Even though this corrosion cannot be eliminated, it can be controlled.
General Corrosion
General corrosion involving water and steel generally results from chemical action where the steel surface oxidizes, forming iron oxide (rust). Many of the systems and components in the plant are made from iron.
Some standard methods associated with material selection that protect against general corrosion include:
- The use of corrosion-resistant materials such as stainless steel and nickel, chromium, and molybdenum alloys. (Keep in mind that the corrosion is electrochemical by nature, and the corrosion resistance of the stainless steels results from surface oxide films that interfere with the electrochemical process.)
- The use of protective coatings such as paints and epoxies.
- The application of metallic and nonmetallic coatings or linings to the surface which protects against corrosion, but allows the material to retain its structural strength (for example, a carbon steel pressure vessel with stainless steel cladding as a liner).
Galvanic Corrosion
Galvanic corrosion occurs when two dissimilar metals with different potentials are placed in electrical contact in an electrolyte. It may also take place with one metal with heterogeneities (dissimilarities) (for example, impurity inclusions, grains of different sizes, difference in composition of grains, or differences in mechanical stress). A difference in electrical potential exists between the different metals and serves as the driving force for electrical current flow through the corrodant or electrolyte. This current results in corrosion of one of the metals. The larger the potential difference, the greater the probability of galvanic corrosion. Galvanic corrosion only causes deterioration of one of the metals. The less resistant, more active one becomes the anodic (negative) corrosion site. The stronger, more noble one is cathodic (positive) and protected. If there were no electrical contact, the two metals would be uniformly attacked by the corrosive medium. This would then be called general corrosion.
For any particular medium, a list can be made arranging metals sequentially from most active, or least noble, to passive, or most noble. The galvanic series for sea water is discussed in the Chemistry Fundamentals Handbook.
Galvanic corrosion is of particular concern in design and material selection. Material selection is important because different metals come into contact with each other and may form galvanic cells. Design is important to minimize differing flow conditions and resultant areas of corrosion buildup. Loose corrosion products are important because they can be transported to the reactor core and irradiated.
In some instances, galvanic corrosion can be helpful in the plant. For example, if pieces of zinc are attached to the bottom of a steel water tank, the zinc will become the anode, and it will corrode. The steel in the tank becomes the cathode, and it will not be effected by the corrosion. This technique is known as cathodic protection. The metal to be protected is forced to become a cathode, and it will corrode at a much slower rate than the other metal, which is used as a sacrificial anode.
Localized Corrosion
Localized corrosion is defined as the selective removal of metal by corrosion at small areas or zones on a metal surface in contact with a corrosive environment, usually a liquid. It usually takes place when small local sites are attacked at a much higher rate than the rest of the original surface. Localized corrosion takes place when corrosion works with other destructive processes such as stress, fatigue, erosion, and other forms of chemical attack. Localized corrosion mechanisms can cause more damage than any one of those destructive processes individually. There are many different types of localized corrosion. Pitting, stress corrosion cracking, chloride stress corrosion, caustic stress corrosion, primary side stress corrosion, heat exchanger tube denting, wastage, and intergranular attack corrosion are discussed in detail in the Chemistry Fundamentals Handbook.
Stress-Corrosion Cracking
One of the most serious metallurgical problems and one that is a major concern in the nuclear industry is stress-corrosion cracking (SCC). SCC is a type of intergranular attack corrosion that occurs at the grain boundaries under tensile stress. It tends to propagate as stress opens cracks that are subject to corrosion, which are then corroded further, weakening the metal by further cracking. The cracks can follow intergranular or transgranular paths, and there is often a tendency for crack branching.
The cracks form and propagate approximately at right angles to the direction of the tensile stresses at stress levels much lower than those required to fracture the material in the absence of the corrosive environment. As cracking penetrates further into the material, it eventually reduces the supporting cross section of the material to the point of structural failure from overload.
Stresses that cause cracking arise from residual cold work, welding, grinding, thermal treatment, or may be externally applied during service and, to be effective, must be tensile (as opposed to compressive).
SCC occurs in metals exposed to an environment where, if the stress was not present or was at much lower levels, there would be no damage. If the structure, subject to the same stresses, were in a different environment (noncorrosive for that material), there would be no failure. Examples of SCC in the nuclear industry are cracks in stainless steel piping systems and stainless steel valve stems.
The most effective means of preventing SCC in reactor systems are: 1) designing properly; 2) reducing stress; 3) removing critical environmental species such as hydroxides, chlorides, and oxygen; 4) and avoiding stagnant areas and crevices in heat exchangers where chloride and hydroxide might become concentrated. Low alloy steels are less susceptible than high alloy steels, but they are subject to SCC in water containing chloride ions. Nickel-based alloys, however, are not effected by chloride or hydroxide ions.
An example of a nickel-based alloy that is resistant to stress-corrosion cracking is inconel. Inconel is composed of 72% nickel, 14-17% chromium, 6-10% iron, and small amounts of manganese, carbon, and copper.
Chloride Stress Corrosion
One of the most important forms of stress corrosion that concerns the nuclear industry is chloride stress corrosion. Chloride stress corrosion is a type of intergranular corrosion and occurs in austenitic stainless steel under tensile stress in the presence of oxygen, chloride ions, and high temperature.
It is thought to start with chromium carbide deposits along grain boundaries that leave the metal open to corrosion. This form of corrosion is controlled by maintaining low chloride ion and oxygen content in the environment and use of low carbon steels.
Caustic Stress Corrosion
Despite the extensive qualification of inconel for specific applications, a number of corrosion problems have arisen with inconel tubing. Improved resistance to caustic stress corrosion cracking can be given to inconel by heat treating it at 620°C to 705°C, depending upon prior solution treating temperature. Other problems that have been observed with inconel include wastage, tube denting, pitting, and intergranular attack.
Hydrogen Embrittlement
Personnel need to be aware of the conditions for hydrogen embrittlement and its formation process when selecting materials for a reactor plant. This chapter discusses the sources of hydrogen and the characteristics for the formation of hydrogen embrittlement.
Concern
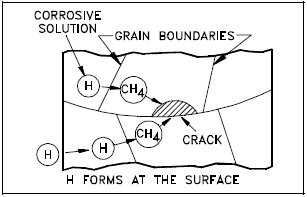
Another form of stress-corrosion cracking is hydrogen embrittlement. Although embrittlement of materials takes many forms, hydrogen embrittlement in high strength steels has the most devastating effect because of the catastrophic nature of the fractures when they occur. Hydrogen embrittlement is the process by which steel loses its ductility and strength due to tiny cracks that result from the internal pressure of hydrogen (H2) or methane gas (CH4), which forms at the grain boundaries. In zirconium alloys, hydrogen embrittlement is caused by zirconium hydriding. At nuclear reactor facilities, the term "hydrogen embrittlement" generally refers to the embrittlement of zirconium alloys caused by zirconium hydriding.
Sources of Hydrogen
Sources of hydrogen causing embrittlement have been encountered in the making of steel, in processing parts, in welding, in storage or containment of hydrogen gas, and related to hydrogen as a contaminant in the environment that is often a by-product of general corrosion. It is the latter that concerns the nuclear industry. Hydrogen may be produced by corrosion reactions such as rusting, cathodic protection, and electroplating. Hydrogen may also be added to reactor coolant to remove oxygen from reactor coolant systems.
Hydrogen Embrittlement of Stainless Steel
As shown in Figure 10, hydrogen diffuses along the grain boundaries and combines with the carbon (C), which is alloyed with the iron, to form methane gas. The methane gas is not mobile and collects in small voids along the grain boundaries where it builds up enormous pressures that initiate cracks. Hydrogen embrittlement is a primary reason that the reactor coolant is maintained at a neutral or basic pH in plants without aluminum components.
If the metal is under a high tensile stress, brittle failure can occur. At normal room temperatures, the hydrogen atoms are absorbed into the metal lattice and diffused through the grains, tending to gather at inclusions or other lattice defects. If stress induces cracking under these conditions, the path is transgranular. At high temperatures, the absorbed hydrogen tends to gather in the grain boundaries and stress-induced cracking is then intergranular. The cracking of martensitic and precipitation hardened steel alloys is believed to be a form of hydrogen stress corrosion cracking that results from the entry into the metal of a portion of the atomic hydrogen that is produced in the following corrosion reaction.
3 Fe + 4 H2O → Fe3O4 + 4 H2
Hydrogen embrittlement is not a permanent condition. If cracking does not occur and the environmental conditions are changed so that no hydrogen is generated on the surface of the metal, the hydrogen can rediffuse from the steel, so that ductility is restored.
To address the problem of hydrogen embrittlement, emphasis is placed on controlling the amount of residual hydrogen in steel, controlling the amount of hydrogen pickup in processing, developing alloys with improved resistance to hydrogen embrittlement, developing low or no embrittlement plating or coating processes, and restricting the amount of in-situ (in position) hydrogen introduced during the service life of a part.
Hydrogen Embrittlement of Zirconium Alloys
Hydrogen embrittlement is a problem with zirconium and zirconium alloys, which often are used as cladding materials for nuclear reactors. Zirconium reacts with water as follows.
Zr + 2 H2O → ZrO2 + 2 H2↑
Part of the hydrogen produced by the corrosion of zirconium in water combines with the zirconium to form a separate phase of zirconium hydride (ZrH1.5) platelets. The metal then becomes embrittled (ductility decreases) and it fractures easily. Cracks begin to form in the zirconium hydride platelets and are propagated through the metal. Zircaloy-2 (a zirconium alloy), which has been used as a fuel rod cladding, may absorb as much as 50% of the corrosion-produced hydrogen and is subject to hydrogen embrittlement, especially in the vicinity of the surface. Studies at Westinghouse, Batelle, and elsewhere have revealed that the nickel in the zircaloy-2 was responsible for the hydrogen pickup. This has led to the development of zircaloy- 4, which has significantly less nickel than zircaloy-2 and is less susceptible to embrittlement. In addition, the introduction of niobium into zircaloy-4 further reduces the amount of hydrogen absorption.
PDH Classroom offers a continuing education course based on this properties of metals reference page. This course can be used to fulfill PDH credit requirements for maintaining your PE license.
Now that you've read this reference page, earn credit for it!





